Selasa, 31 Oktober 2017
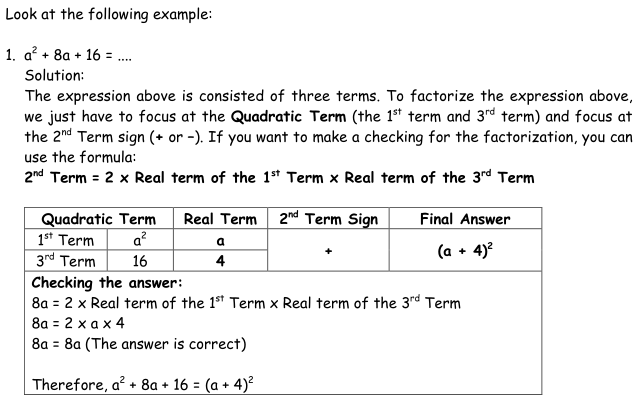
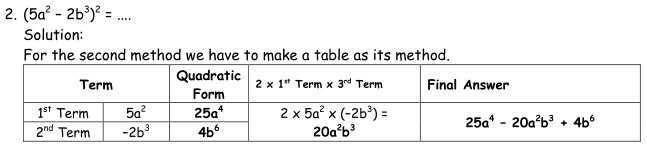
Selasa, 24 Oktober 2017
Garis - garis istimewa pada segitiga
Garis-garis istimewa pada sebuah segitiga adalah sebagai berikut:
Garis tinggi
Garis tinggi adalah sebuah garis pada segitiga yang ditarik dari salah satu sudut pada segitiga dan tegak lurus terhadap sisi dihadapannya.
Perhatikan gambar di bawah ini.

Gambar 1: Garis-garis tinggi pada segitiga lancip.
AD, BE dan CF adalah garis-garis tinggi segitiga lancip ABC.
Gambar 2: Garis-garis tinggi pada segitiga tumpul.
AD, BE dan CF adalah garis-garis tinggi segitiga tumpul ABC.
Gambar 3: Garis-garis tinggi pada segitiga siku-siku.
AB, BE dan BC adalah garis-garis tinggi segitiga siku-siku ABC. Garis berat
Garis berat adalah sebuah garis yang ditarik dari salah satu sudut pada segitiga dan membagi sisi dihadapannya menjadi dua sama besar.
Perhatikan gambar di bawah ini.

Gambar 4: Garis-garis berat
AD, BE dan CF adalah garis-garis berat segitiga ABC. Perhatikan bahwa garis-garis berat ini membagi sisi di hadapan masing-masing sudut menjadi dua sama besar.
Garis sumbu
Garis sumbu adalah sebuah garis yang membagi dua sebuah sisi pada segitiga dan tegak lurus terhadap sisi tersebut.
Perhatikan gambar di bawah ini.

Gambar 5: Garis-garis sumbu segitiga ABC
Garis bagi
Garis bagi adalah sebuah garis yang membagi dua sebuah sudut pada segitiga sama besar.
Perhatikan gambar di bawah ini.

Gambar 5: Garis-garis bagi segitiga ABC
AD, BE dan CF adalah garis-garis bagi segitiga ABC. Perhatikan bahwa garis-garis bagi ini membagi masing-masing sudut menjadi dua sama besar.
-Selamat belajar, Tuhan memberkati-
Angles Relationship (Angles Word Problems)
Few problems about angles relationship are given in a word problems.
Problem 1.
The complement of an angle is 56º. What is the measure of the angle?
Solution:
Assumed: The angle is x, then its complement is 90º - x.
The mathematics model of "The complement of an angle is 56º" is:
90º - x = 56º
-x = 56º - 90ºº
-x = -34º
x = 34ºº
Then the measure of the angle is 34º.
Problem 2.
The magnitude of an angle is 5 times of its supplement. What is the magnitude of the angle?
Solution:
Assumed: The angle is x, then its supplement is 180º - x.
The mathematics model of "The magnitude of an angle is 5 times of its supplement" is:
x = 5(180º - x)
x = 900º - 5x
x + 5x = 900º
6x = 900º
x = 150º
Then the magnitude of the angle is 150º.
Problem 3.
The complement of an angle is a quarter of the supplement of the angle. What is the 5 times of the angle?
Solution:
Assumed the angles is x, then
the complement is 90º - x and
its supplement is 180º - x.
4(90º - x) = 180º - x
360º - 4x = 180º - x
-4x + x = 180º - 360º
-3x = -270º
3x = 270º
x = 90º
Then 5 times of the angle = 5.90º = 450º.
Problem 4.
The supplement of twice (2 times) of the complement of an angle is 80º. What is 1/4 of the angle?
Solution:
Assume: angle = x
The complement = 90º - x
2 times of the complement = 2 (90º – x)
Supplement of 2 times of the complement = 180º - 2 (90º - x) = 80º
The complement = 90º - x
2 times of the complement = 2 (90º – x)
Supplement of 2 times of the complement = 180º - 2 (90º - x) = 80º
180º - 2 (90º - x) = 80º
180º - 180º + 2x = 80º
2x = 80º
x = 40º
Therefore 1/4 of the angle = 1/4 . 40º = 10º
Therefore 1/4 of the angle = 1/4 . 40º = 10º
The complement of 1/2 supplement of 1/4 of an angle is 20º. Determine the magnitude of the angle!
Solution:Assume: angle = x
1/4 of angle = 1/4 x
supplement of 1/4 of angle = 180º - 1/4 x
1/2 supplement 1/4 of angle = 1/2 (180º - 1/4 x)
complement of 1/2 of supplement of 1/4 angle:
90º - 1/2 (180º - 1/4 x) = 20º (open the brackets)
90º - 90º + 1/8 x = 20º
1/8 x = 20º
x = 160º
Therefore the magnitude of the angle is 160º.
-Vn-
Langganan:
Postingan (Atom)